Mapping the structure of a glass through its voids

What happens to vitreous silica v-SiO₂, the ubiquitous glass in nature, when it is compressed? Its density of course increases, but the real challenge is the description of this process down to the atomic lengthscale. X-ray diffraction (XRD) and positron lifetime annihilation spectroscopy (PALS) were exploited to map the structure of a set of permanently densified SiO₂ glasses. They provide a picture of silica as a porous medium where about 20 % of the volume is occupied by sub-nano voids whose density variation dominates both the compressibility and the medium range order up to densities close to that of α-quartz.

Glasses are part of the wide family of disordered materials. This means that their structure lacks any long-range order even if it is far from being totally random [1]. First-neighbor atoms are often disposed in well-defined arrangements and almost ordered structures persist up to a few interatomic distances, leading to the so-called medium range order (MRO). The MRO is revealed by neutron or x-ray diffraction through the appearance of a first sharp diffraction peak (FSDP) in the measured static structure factor S(Q). However, though it appears fundamental in the comprehension of glass phenomenology, a precise microscopic view of the MRO characteristic length scale still proves elusive.

Vitreous silica is characterized by a disordered open network formed by SiO₄ tetrahedra. Permanent densification was achieved by using a high-pressure, high-temperature technique [2,3]. Samples were prepared under different pressures and cover a density range from that of normal silica (2.20 g/cm³) up to a density 22% higher than that (2.67 g/cm³). Although subjected to a relevant density change, XRD shows that the SiO4 tetrahedra are basically unaffected. This means that the main effects of densification need to be sought for in-between the tetrahedra, namely in the interstitial void spaces.

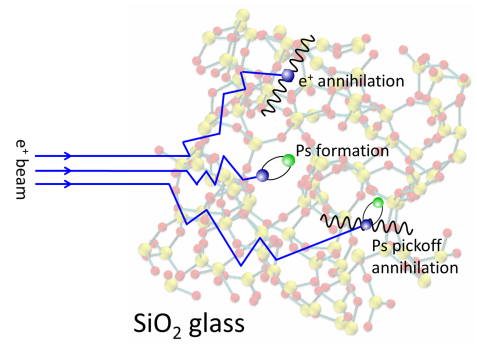
PALS represents an unique tool for looking into the interstitial voids in glasses and mapping their evolution as a function of density. The main observable of a PALS experiment is the time that elapses between the positron e+ implantation and its annihilation. Different times correspond to different annihilation channels and these give information on the nature of the sample, see Fig. 1. In an insulator with open volumes, e+ can also form positronium (Ps), an e⁺-e^– bound state. Ps exists in two spin states: the singlet state para-positronium (pPs, lifetime in vacuum τp=125 ps) and the long living triplet state ortho-positronium (oPs, lifetime in vacuum τo=142 ns). In condensed matter, the latter lifetime is reduced via pickoff annihilation with the electron cloud limiting the void volume. However, the pickoff lifetime is still longer than that of the other annihilation processes and it conveys information on the void dimensions.
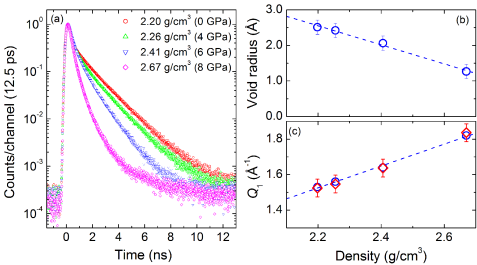
Experiments were performed at the pulsed low energy positron system (PLEPS), fed by the NEPOMUC positron source of the FRM II reactor (Garching, Germany). PALS spectra were acquired at two implantation energies of 16 and 18 keV that correspond to average implantation depths ranging between 1 and 2 μm. An example of PALS data is reported in Fig. 2(a).

The analysis performed with PATFIT shows the presence of three main lifetime components: (i) a short lifetime τ₁ which comes from pPs and e⁺ annihilation in bulk silica; (ii) an intermediate lifetime τ₂ probably due to oPs or e⁺ annihilation in small voids or vacant sites; (iii) a long lifetime τ₃ that is related to oPs pickoff in the intrinsic structural voids. The decrease in this longest decaying lifetime going from normal silica to the sample of highest density is evident from Fig. 2(a) and is related to the shrinking of the intrinsic voids in the silica samples. Being more quantitative, the oPs pick-off lifetime can be related to the interstitial voids dimension according to the Tao-Eldrup model [4,5]. We find that, on increasing the density by 22 %, the void radius shrinks linearly to half of its initial value, Fig. 2(b). Moreover, the intensity of the third lifetime component is also reduced, thus implying a diminution of the number of voids.
These results naturally suggest a description of v-SiO₂ as a porous medium whose structure is made up of an incompressible and a compressible part, namely SiO₄ tetrahedra and interstitial voids, respectively. In normal silica, the compressible part occupies ∼18% of the total volume. Upon densification, the tetrahedra rotate almost rigidly to fill the interstitial voids so that the compressible part is reduced to ∼3 % of its initial value [2].

The densification process also induces a modification in the MRO. With increasing density, the FSDP position Q1 shifts upwards by about 20 %. This behavior can be explained in terms of a reduction in the volume of the voids as well.
The vitreous silica network can be further described as an ensemble of voids surrounded, at a certain distance D from their centers, by quasi-spherical cation-centered clusters, i.e. SiO₄ tetrahedra separated by the Si-Si distance d. The void-cluster distance D can be view as the sum of the PALS void radius R and a screening distance depending on the tetrahedral structure. The two distances D and d are the main ingredients of the so-called void-cluster model for the FSDP [6]. Fig. 2© shows how PALS data allow us to describe with great accuracy both the FSDP position and its density dependence in absolute units, shedding some new light on the subtle nature of MRO [2].